Cross products have various applications that are very important in the real world.
In this article, you will learn to use the right hand rule for cross product through some examples.
If A and B are taken as two independent vectors, then the cross product of these two vectors (AB) will be perpendicular to both the vectors, and it will be normal to the plane having both vectors. It can be represented as-.
a . b = ||a|| ||b|| cos (θ)
The critical thing to remember is that the result is a vector and NOT a scalar value. This is why it is known as vector product. For the definition easy to remember, we generally use determinants to calculate cross products and we made a cross product calculator that helps you in finding the cross product of two vectors.
To calculate the position between vectors we also use dot product and the difference between cross product and dot product is the result of cross product will be vector quantity while the result of dot product will be scalar quantity.
Right Hand Rule For Cross Product
In maths and physics, the right hand rule is a standard illustration for assimilating notation conventions for vectors in 3-D. It was for the first time introduced by John Ambrose Flemming in the late 19th century.
As I already said, there are two noticeable solutions for vectors at right angles to each other. So, while using this idea, one must remove the ambiguity of which solution is meant.
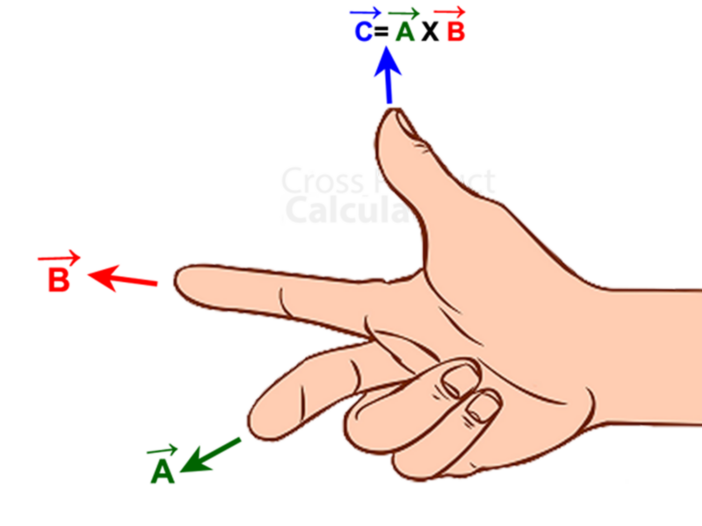
One type of right hand rule is used while performing an ordered operation on two vectors, let it be “a” and “b”. And the result of this is a vector “c” that is perpendicular to each “a” and “b”.
So to impose the right hand rule, you should follow the below-given instruction to choose one of the two directions.
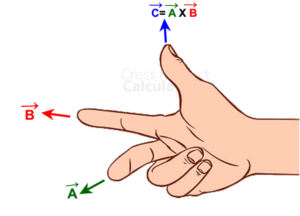
- Hold up your right hand. Taking the left hand won’t work.
- Arrange your thumb, index and middle finger at right angles to each other in a way that your index finger should be pointed straight.
- The middle finger should be in the direction of vector product . Here, the thumb will represent vector “a”, and the index finger will represent vector “b”.
- Nevertheless, interchangeable finger assignments are also possible.
Another form of right-hand rule is taken into account when a vector is assigned for the rotation of the body or a magnetic field. Conversely, if a vector specifies any rotation and you have to find how the cycle occurs. Then, this second form of right hand rule, also called the right-hand grip rule, comes into use in such cases.
- Curl fingers of your right hand (hence the name) in a way that follows the rotation of vectors “a” and “b”. Then your thumb will point towards the vector product that is “c”.
This method is taken into consideration when you have to find the direction of the torque vector. When you grip the imaginary axis of the rotation due to a rotational force, your fingers will show the guide of the force, and your extended thumb will point in the direction of the torque vector.
Right Hand Rule For Cross Product
Its highly useful to know the cross product between the distinct unit vectors , and .
For instance, consider
×
Here, because the vectors are perpendicular or orthogonal, their magnitude will be-
= = (1) (1) = 1
Now because the unit vectors have magnitude 1. The cross product has to be straight-up or perpendicular to and
This suggests that it must point along the z-axis. And because it has length 1, it can be either or .
How to decide which one it is? Well, it’s arbitrary. We can choose any of the two options, and it will work the same way in mathematics.
But, of course, it will be constructive if we all agree on the same result.
We have decided this, and this particular choice is called a right-handed coordinate system.
So, if you have to choose a coordinate system for a problem, then make it a right-hand coordinate system or else there will be a chance of getting incorrect answers.
Some Applications of Right Hand Rule
- It is used to ascertain the direction of the cross or vector products.
- To find the torque and force that causes it.
- Also, it can be used to find the position of the application of the force that’s causing it.
- It can be helpful in magnetic fields to determine the position of the electric current
- To find the magnetic field force on charged particles and for determining the velocity of the object.
Make sure to practice the right-hand rule for cross-product after reading this article.
